Home >> PURE MATHS, Algebra, partial fractions

Some Definitions:

Proper Fraction

When the degree(index) of the function is higher in the denominator than the numerator.

Improper Fraction

When the degree(index) of the function is higher in the numerator than the denominator.

Partial Fractions

Factorising the denominator of a proper fraction means that the fraction can be expressed as the sum(or difference) of other proper fractions.

Simple Addition/Subtraction of Algebraic Fractions

As with simple fraction arithmetic, a common denominator is found from the denominators of either fraction.

The numerators are subsequently altered to be fractions of the new denominator.

back to top

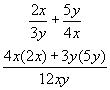
Equations & Identities

Equations are satisfied by discrete values of the variable involved.

Example:

Identities are satisfied by any value of the variable used.

Note the equals sign '=' is modified to reflect this.

Example:

When we make partial fractions(below) we are creating an identity from the original expression.

back to top

Denominator with only 'Linear Factors'

By 'linear' we mean that x has a power no higher than '1' . In other words, this method does not work with x², x³, x⁴ etc.

For each linear factor of the type:

there is a partial fraction:

hence:

where x is a variable and A,B,a,b,c,d are constants, where 'a' is not equal to 'b'.

Example #1

back to top

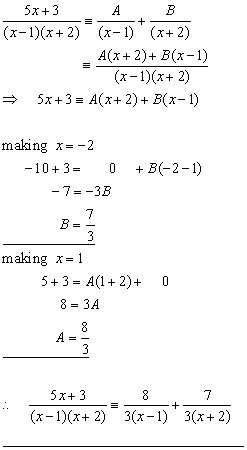
Denominator with 'Repeated Linear Factors

For each 'repeated' linear factor of the type:

there is a partial fraction:

hence:

where x is a variable and A,B,C,a,b,c,d are constants, where 'a' is not equal to 'b'.

Example #1

back to top

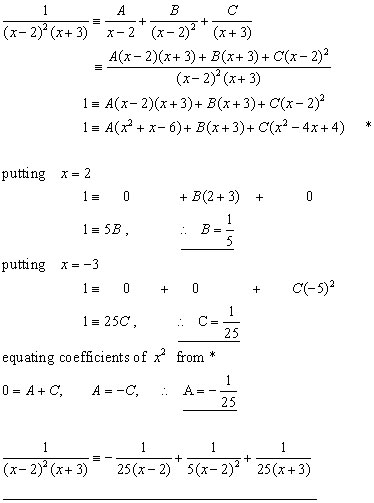
Denominator with a Quadratic Factor

For each quadratic factor of the type:

there is a partial fraction:

hence:

Example #1

back to top

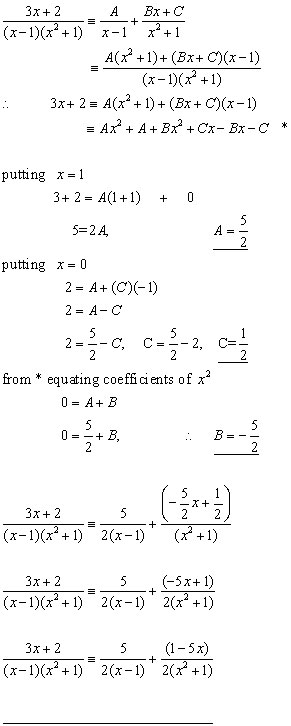
[ About ] [ FAQ ] [ Links ] [ Terms & Conditions ] [ Privacy ] [ Site Map ] [ Contact ]