Home >> PURE MATHS, Integration, differential equations
introduction |
Introduction
All equations with derivatives of a variable w.r.t. another are called 'differential equations'.
A first order differential equation contains a first derivative eg dy/dx.
It might not be appreciated, but ALL integrals are derived from original 'first-order' differential equations.
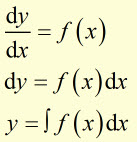
Example:
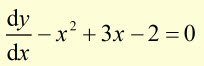Write y as a simple function of x.
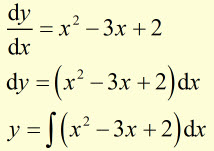
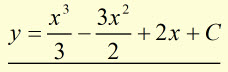
(where C is the constant of integration)
First Order with 'Variables Separable'
Solution is by collecting all the 'y' terms on one side, all the 'x' terms on the other and integrating each expression independently.
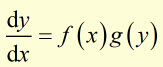
rearranging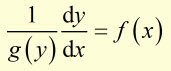 
integrating both sides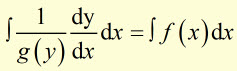 
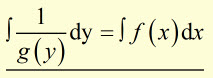
Example #1
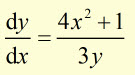
rearranging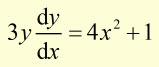 
integrating both sides
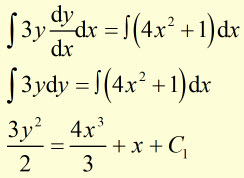
multiplying by 6
dividing by 9
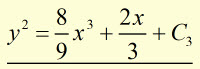
Note how the constant of integration C changes its value.
Example #2
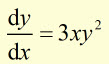
rearranging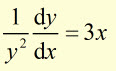 
integrating both sides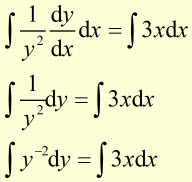 
multiplying both siides by 2y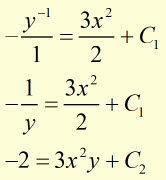 
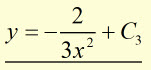
First Order 'linear' differential equations
By definition 'linear' differential equation have the form:
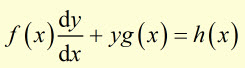
Dividing by f(x) to make the coefficient of dy/dx equal to '1', the equation becomes:
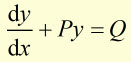
(where P and Q are functions of x, and only x)
The key to solving these types of problem is to choose a multiplying factor(sometimes called an 'integrating factor').
This is to make the LHS of the equation appear like a result from the Product Rule.
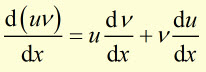
Example Find the P and Q functions and for the following differential equation, expressing it in terms of x and y only.
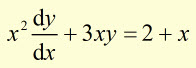
rearranging,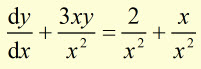 
cancelling x's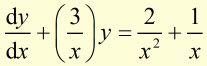 
(i
recalling that,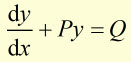
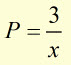
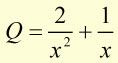
multiplying equation (i by 
to make the LHS like a Product Rule result,
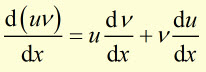
where 
and 
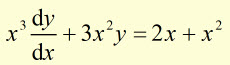(ii
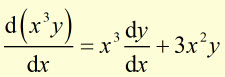
from the equality (ii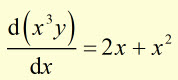 
integrating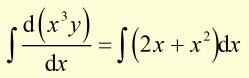 
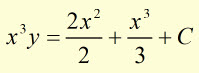
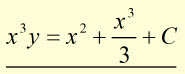
this week's promoted video
[ About ] [ FAQ ] [ Links ] [ Terms & Conditions ] [ Privacy ] [ Site Map ] [ Contact ]